Mermin pentagrams, Cayley-Salmon, Desargues

 

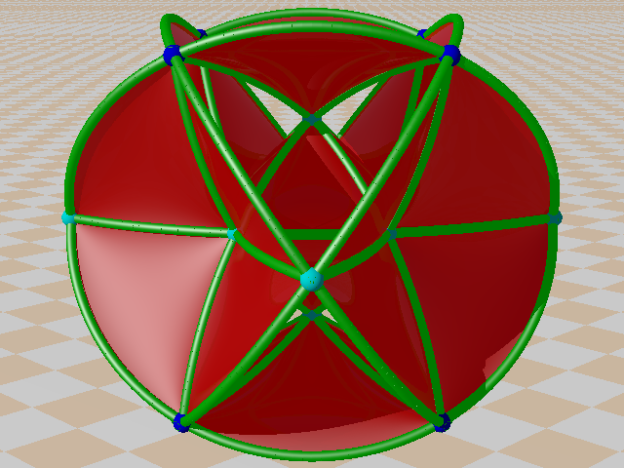
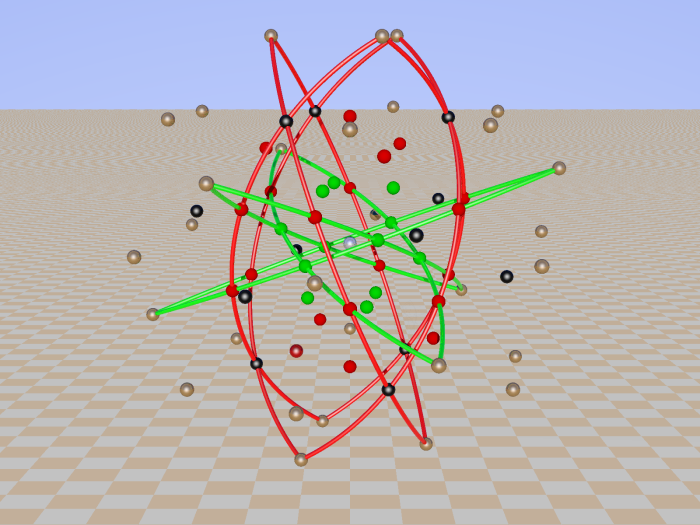
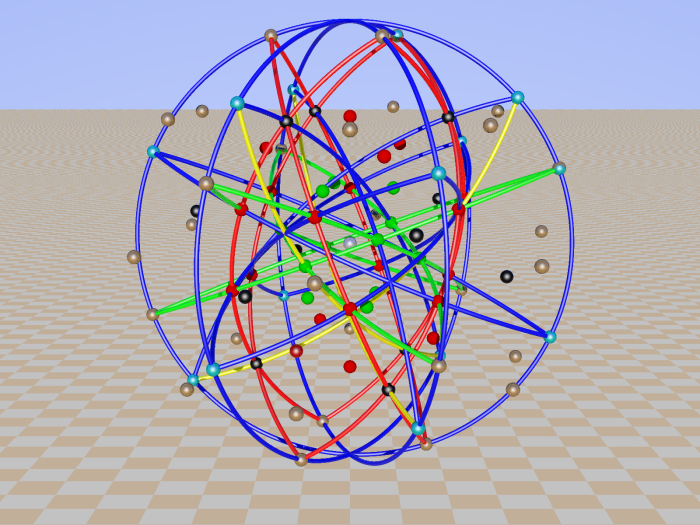
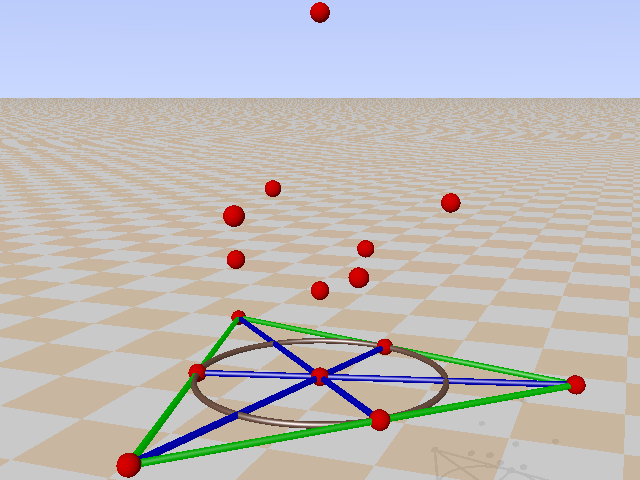
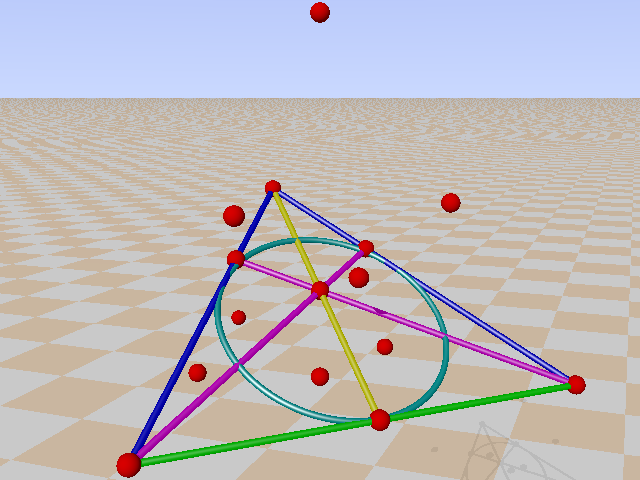
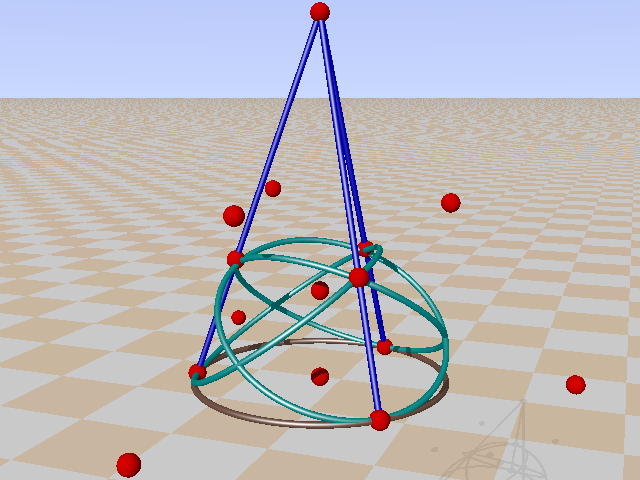
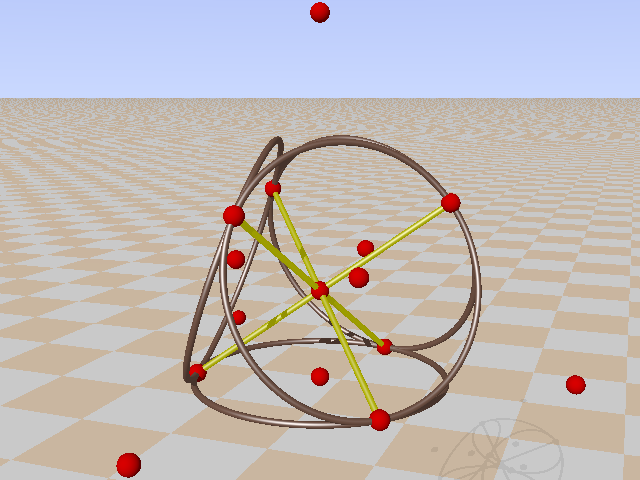
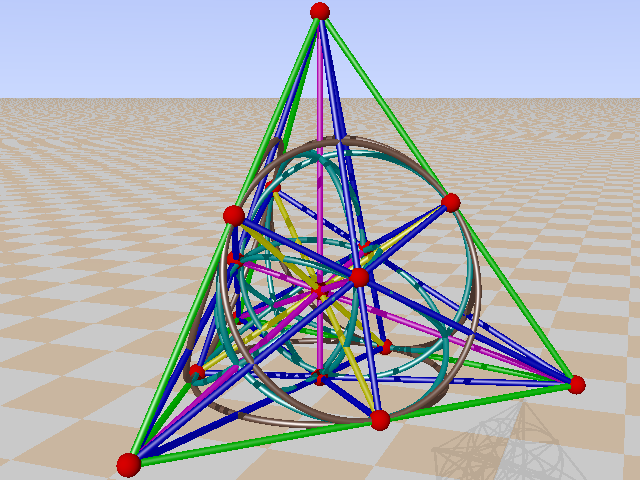
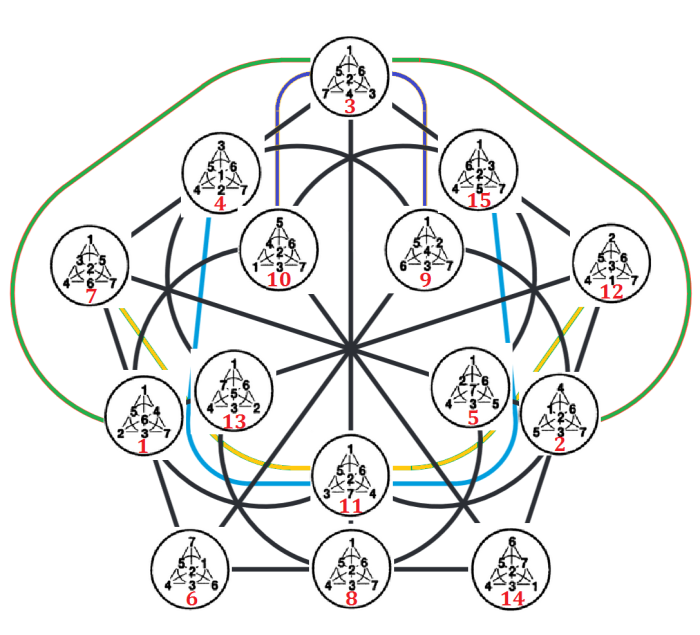
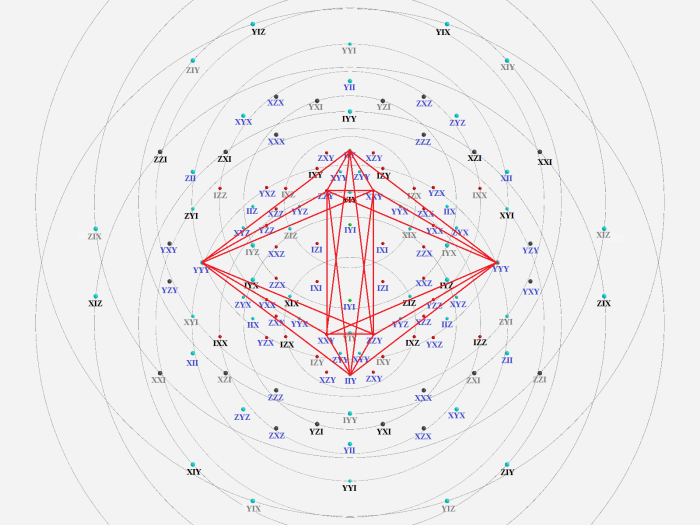
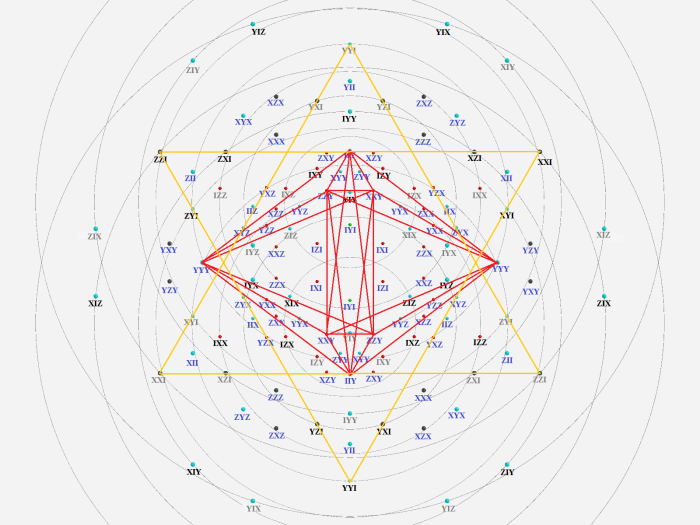
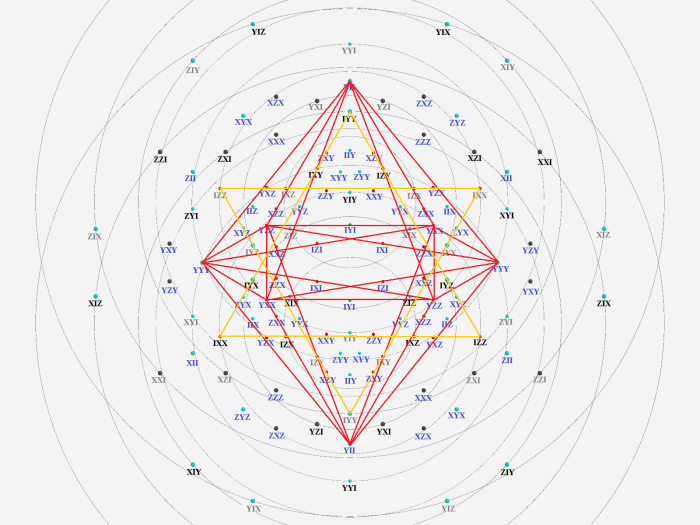
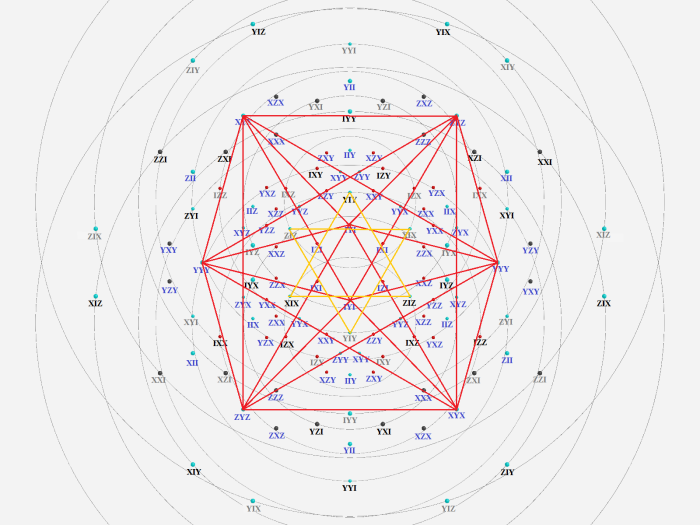
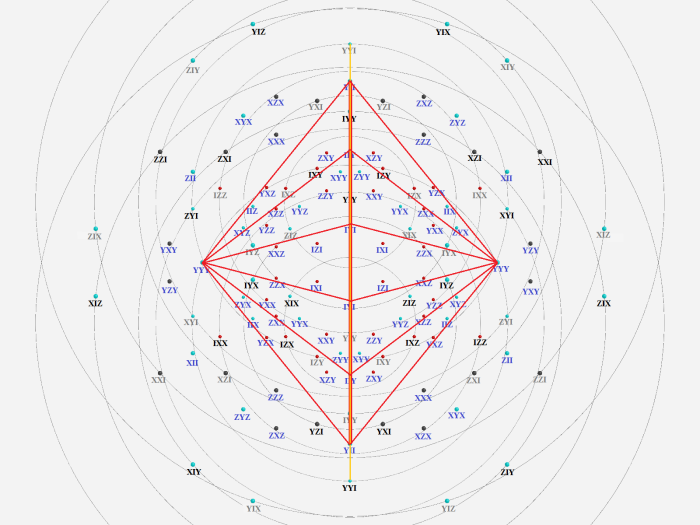
In the Cayley-Salmon configuration, complement of GQ(2,2), one can trace six configurations equivalent to a Mermin pentagram. Points on the Klein quadric are lines in PG(3,2).

FIGURE 1

In the next 6 pictures they are selected:

FIGURE 2

FIGURE 3

FIGURE 4

FIGURE 5

 

FIGURE 6

I

FIGURE 7

 

 

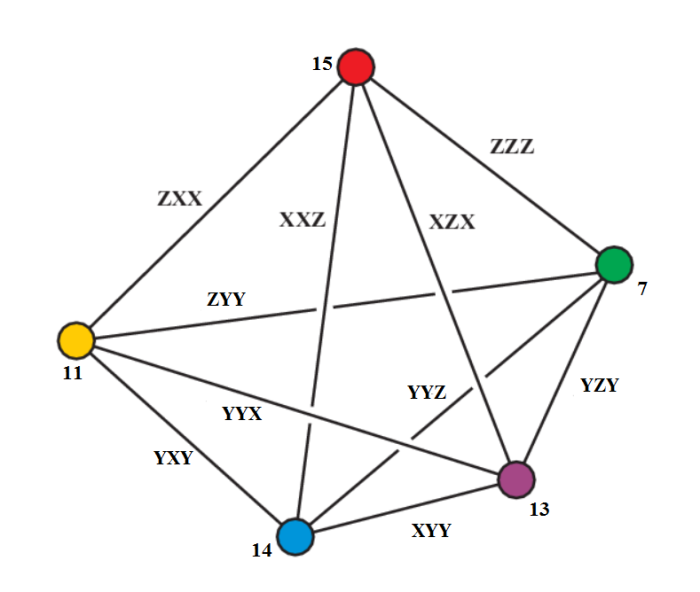
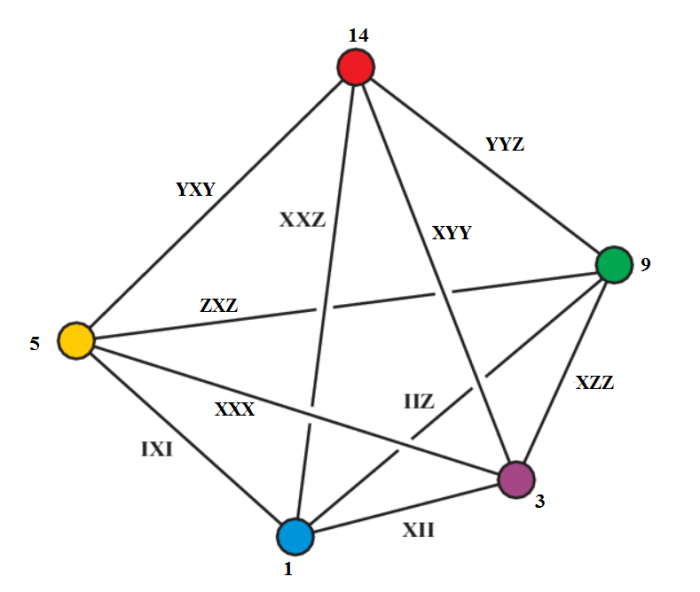
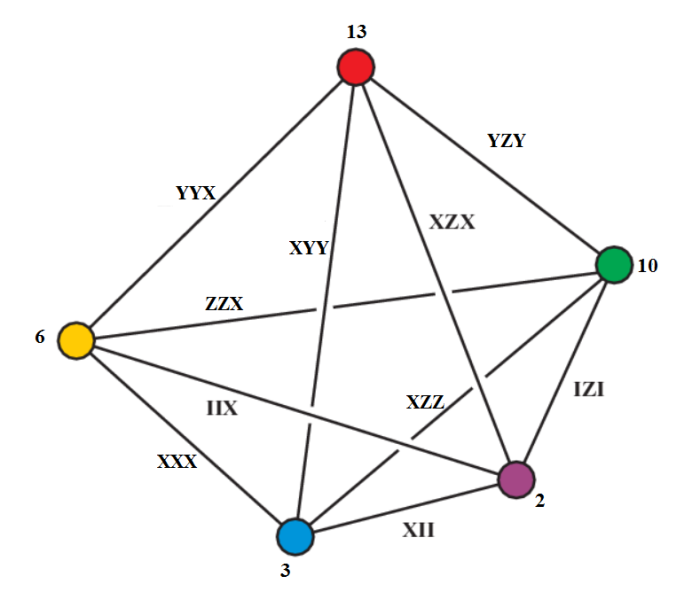
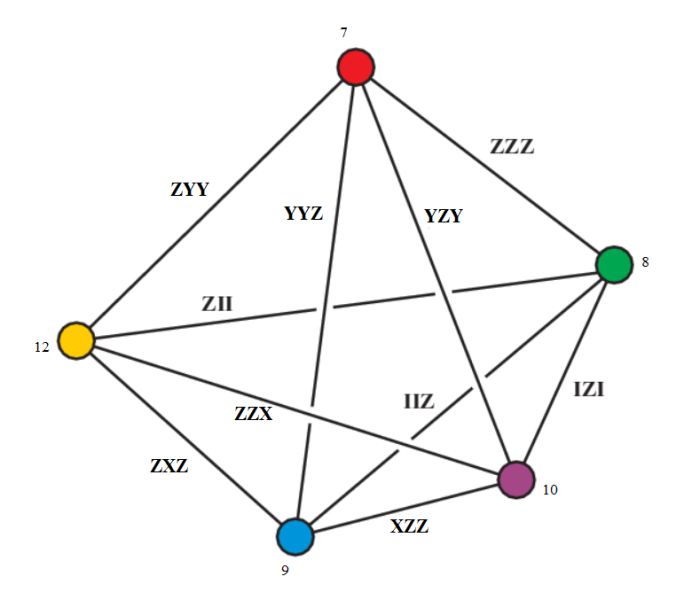
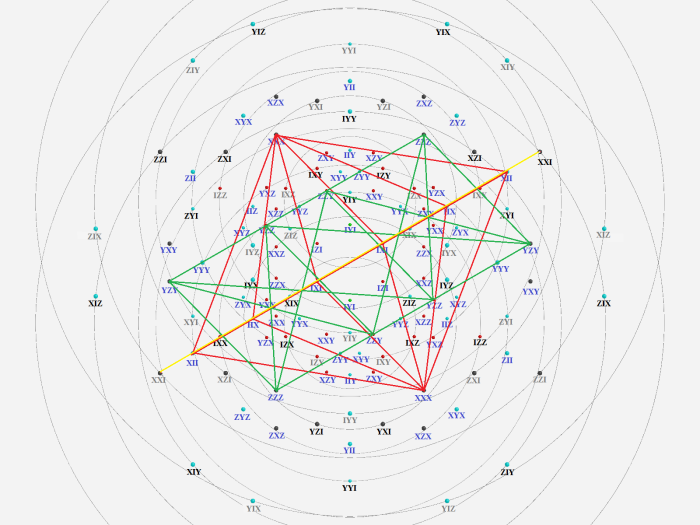
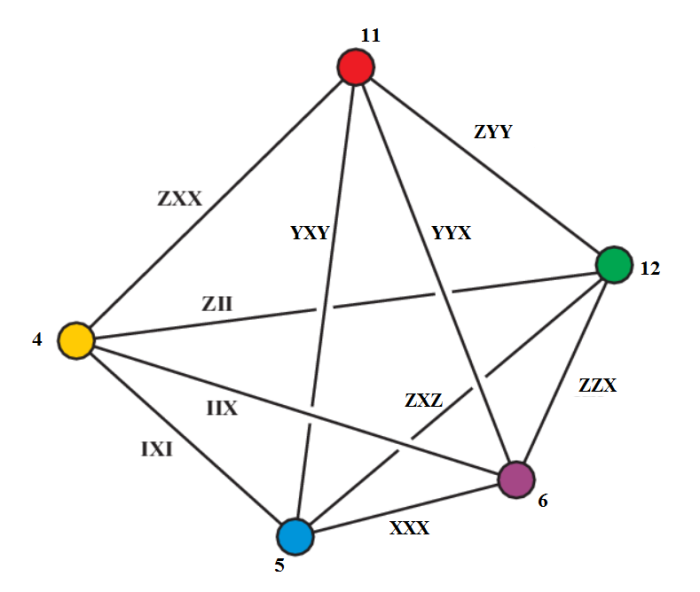
If one choose for planes with four lines instead of pencils of four lines we get Desargues configurations, complement of Petersen graph. Three examples:

 

FIGURE 8   Desargues configuration with the planes 1, 2, 4, 8 and 15

 

 

FIGURE 9  Desargues configuration with planes 7, 11, 13, 14 and 15

 

 

FIGURE 10  Desargues configuration with planes 7, 8, 9, 10 and 12

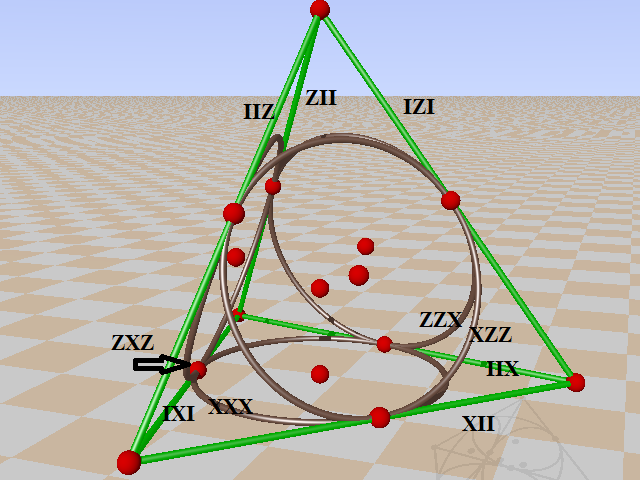
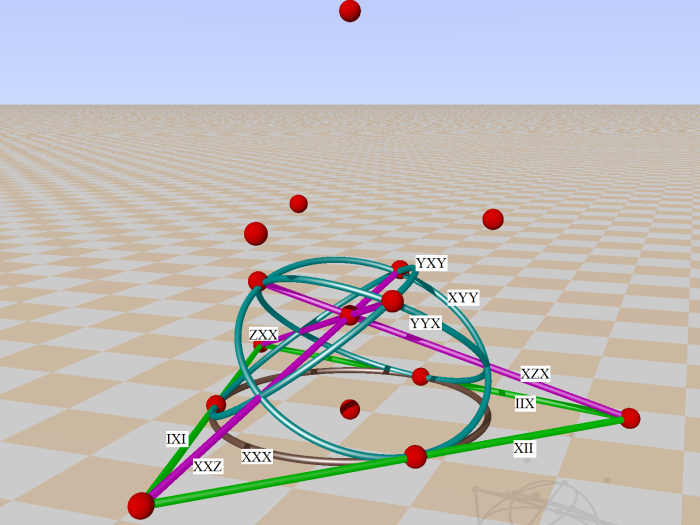
By symmetry you can find the remaining three from the the last one.

Levay and Szabo discuss the Magic Veldkamp Line (MVL) in

Mermin pentagrams arising from Veldkamp lines for three qubits

The double six of Mermin pentagrams that occurs there is just the six pencils and six planes above.

The last picture for instance shows five planes with four lines

XXX XII IXI IIX

XXX XYY YXY YYX

IXI YXY ZXX XXZ

YYX ZXX XZX IIX

XYY XII XZX XXZ

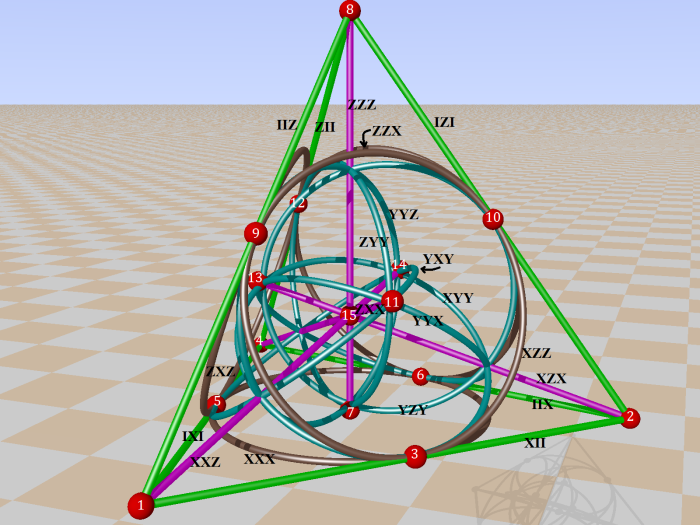
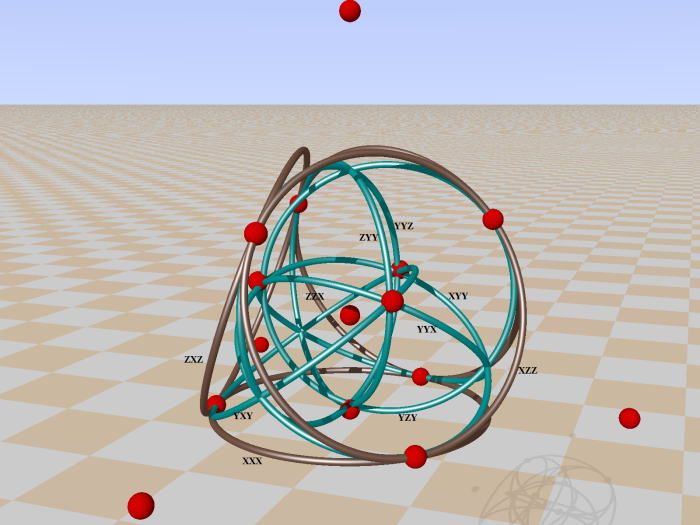
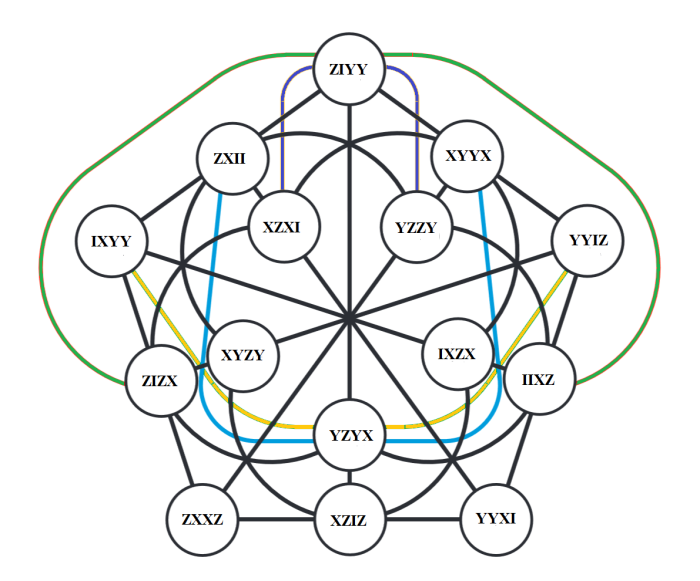
The five planes in PG(3,2) are the 5 lines of a Mermin pentagram. The core of the MVL is made up of 15 elements that are the lines of the GQ below:

 

FIGURE 11

 

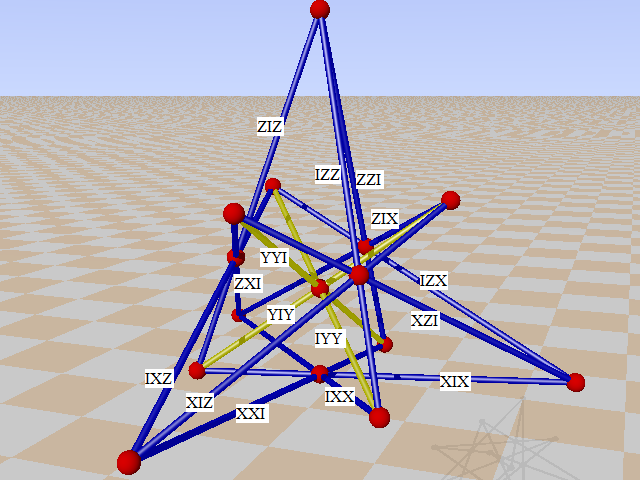
The Lagrangian subspaces correspond to maximal sets of pairwise commuting three-qubit Pauli operators. They are fano planes. They are found by completing the planes in PG(3,2) with the flat pencils of the GQ.

XXX XII IXI IIX          IXX XIX XXI

XXX XYY YXY YYX     IZZ  ZIZ  ZZI

IXI YXY ZXX XXZ       XIZ  ZIX  YIY

YYX ZXX XZX IIX       XZI  ZXI  YYI

XYY XII XZX XXZ       IZX  IXZ  IYY

Spreads in the points of the GQ in PG(5,2) correspond to ovoids in PG(3,2). Call the six ovoids of the GQ A,B,C,D,E,F. Then mark the points by membership of the ovoids.

FIGURE 12

 

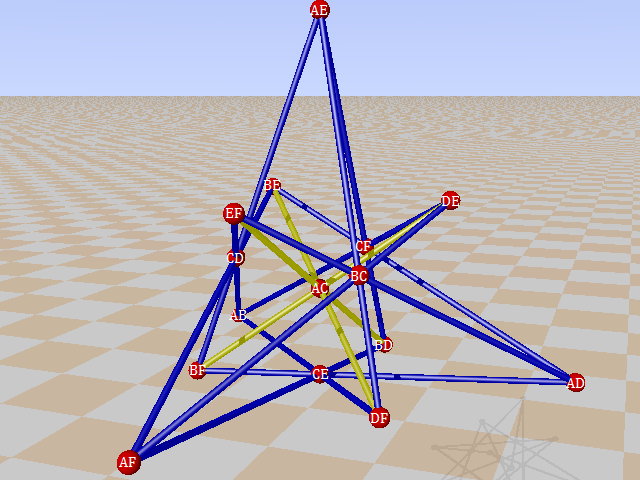
We see that the five added flat pencils are pencils through the five points of ovoid E.

This is the Mermin pentagram with flat pencils through points of ovoid A (FIGURE 9):

YZY ZZX ZYY YYX     XIZ IXZ XXI   AF

YZY XZZ XYY YYZ     ZIX IXX ZXI   AB

ZXZ ZZX XZZ XXX     YIY IYY YYI   AC

ZXZ ZYY YXY YYZ     XIX IZX XZI   AD

XXX XYY YXY YYX    ZIZ  IZZ ZZI   AE

This is the Mermin pentagram with flat pencils through points of ovoid B:

YZY XZZ XYY YYZ     ZIX IXX ZXI   AB

XII  XZZ   IZI   IIZ      XIZ IZZ XZI    BC

XXZ ZZZ YYZ  IIZ      YYI ZZI XXI    BD

XYY XII XZX XXZ      IZX IYY IXZ    BE

YZY XZX IZI ZZZ       YIY XIX ZIZ    BF

This is the Mermin pentagram with flat pencils through points of ovoid C (FIGURE 8):

XII  XXX  IXI   IIX     XIX IXX XXI   CE

ZXZ XXX XZZ ZZX    YIY IYY YYI   AC

XII  XZZ   IZI   IIZ     XIZ  IZZ XZI   BC

ZXZ  IXI   ZII   IIZ     ZIZ  IXZ ZXI   CD

ZZX   IZI   ZII  IIX     ZIX  IZX ZZI    CF

This is the Mermin pentagram with flat pencils through points of ovoid D:

ZXZ ZYY YXY YYZ     XIX IZX XZI   AD

ZXZ  IXI   ZII   IIZ      ZIZ IXZ ZXI   CD

ZII  ZXX ZZZ ZYY      IXX IYY IZZ   DF

XXZ ZZZ YYZ  IIZ      YYI ZZI XXI   BD

IXI YXY ZXX XXZ      YIY XIZ ZIX   DE

This is the Mermin pentagram with flat pencils through points of ovoid F:

YZY ZZX ZYY YYX     XIZ IXZ XXI   AF

ZZX   IZI   ZII   IIX     ZIX  IZX ZZI   CF

ZII   ZXX  ZZZ ZYY    IXX  IYY IZZ   DF

YZY XZX  IZI  ZZZ     YIY  XIX ZIZ   BF

YYX ZXX XZX  IIX     XZI  ZXI YYI   EF

The six pentagrams of the PG(3,2)-pencils of figure 2 to 7 are conjugate to the six pentagrams of the PG(3,2)-planes. I will write down the twelve pentagrams and the 15 flat pencils occuring twice in 2 ovoids. The ovoids in the middle and the conjugate pentagrams at the left and right.

A ( {7-11-13-14-15} figure 9,  [1-2-4-8-15] figure 2 ):

XXXI  {14}   YZY ZZX ZYY YYX     XIZ IXZ XXI     IXI  XXZ  XII  IIZ      [1]   IIIZ

XIXX  {11}   YZY XZZ XYY YYZ     ZIX IXX ZXI     IXI  ZXX  ZII  IIX      [4]   IZII

XXXX {15}   ZXZ ZZX XZZ XXX     YIY IYY YYI     XZX XXZ ZXX ZZZ   [15] ZZZZ

XXIX  {13}   ZXZ ZYY YXY YYZ     XIX IZX XZI     XZX  XII  IZI  IIX      [2]   IIZI

IXXX  {7}     XXX XYY YXY YYX    ZIZ  IZZ ZZI     ZZZ   ZII  IZI  IIZ      [8]   ZIII

B ( {4-5-6-11-12} , [4-5-6-11-12] figure 7 ):

XIXX  {11}   YZY XZZ XYY YYZ     ZIX IXX ZXI     IXI  ZXX  ZII  IIX     [4]    IZII

IXII    {4}     XII  XZZ   IZI   IIZ      XIZ IZZ XZI     ZII  ZXX  IXI  IIX      [11] ZIZZ

IXXI   {6}     XXZ ZZZ YYZ  IIZ      YYI ZZI XXI     ZZX XXX IIX YYX     [6]   IZZI

XXII  {12}   XYY XII XZX XXZ      IZX IYY IXZ     ZII ZYY ZXZ ZZX       [12] ZZII

IXIX  {5}     YZY XZX  IZI ZZZ      YIY XIX ZIZ     IXI ZXZ YXY XXX      [5]   IZIZ

C ( {1-2-4-8-15} figure 8,  [7-11-13-14-15] figure 3 ) :

XIII   {8}     XII  XXX  IXI   IIX     XIX IXX XXI     ZYY ZZZ YZY YYZ      [7]  IZZZ

XXXX {15}  ZXZ XXX XZZ ZZX    YIY IYY YYI      XZX ZZZ ZXX XXZ    [15] ZZZZ

IXII    {4}     XII  XZZ   IZI   IIZ     XIZ IZZ XZI      ZYY ZXX YXY YYX    [11] ZIZZ

IIXI   {2}     ZXZ  IXI   ZII   IIZ     ZIZ IXZ ZXI     XZX YZY XYY YYX      [13] ZZIZ

IIIX   {1}     ZZX   IZI   ZII  IIX     ZIX IZX ZZI     XXZ YXY XYY YYZ      [14] ZZZI

D ( {2-3-6-10-13},  [2-3-6-10-13] figure 5 ):

XXIX {13}  ZXZ ZYY YXY YYZ     XIX IZX XZI     XZX  XII   IZI   IIX       [2]   IIZI

IIXI   {2}    ZXZ  IXI   ZII   IIZ      ZIZ IXZ ZXI     XZX YZY XYY YYX       [13] ZZIZ

IIXX  {3}    ZII  ZXX ZZZ ZYY      IXX IYY IZZ     XYY XZZ XXX   ZII       [3]   IIZZ

IXXI  {6}    XXZ ZZZ YYZ  IIZ      YYI ZZI XXI     ZZX XXX  IIX  YYX       [6]   IZZI

XIXI  {10}  IXI YXY ZXX XXZ     YIY XIZ ZIX      YZY  IZI  XZZ ZZX        [10] ZIZI

E ( {7-8-9-10-12},  [7-8-9-10-12] figure 6):

XIII   {8}   XXX  XII  IXI  IIX       IXX XIX XXI      ZZZ ZYY YZY YYZ        [7]  IZZZ

IXXX {7}   XXX XYY YXY YYX     IZZ  ZIZ  ZZI     ZZZ   ZII  IZI   IIZ        [8]   ZIII

XIXI {10}  IXI YXY ZXX XXZ      XIZ  ZIX  YIY     YZY IZI  XZZ ZZX        [10] ZIZI

XIIX  {9}   YYX ZXX XZX IIX       XZI  ZXI  YYI     IIZ XZZ XZX YYZ        [9]   ZIIZ

XXII  {12} XYY XII XZX XXZ       IZX  IXZ  IYY     ZII ZYY ZXZ ZZX        [12] ZZII

F ({1-3-5-9-14},  [1-3-5-9-14] figure 4 ):

XXXI {14} YZY ZZX ZYY YYX     XIZ IXZ XXI     IXI  XXZ   XII   IIZ         [1]   IIIZ

IIIX   {1}   ZZX   IZI   ZII   IIX     ZIX IZX ZZI     XXZ YXY XYY YYZ         [14] ZZZI

IIXX  {3}   ZII   ZXX  ZZZ ZYY    IXX IYY IZZ     XYY XZZ XXX  XII          [3]   IIZZ

IXIX  {5}   YZY XZX  IZI  ZZZ     YIY XIX ZIZ     IXI  ZXZ YXY XXX          [5]   IZIZ

XIIX  {9}   YYX ZXX XZX  IIX     XZI ZXI YYI     IIZ  XZZ ZXZ YYZ           [9]   ZIIZ

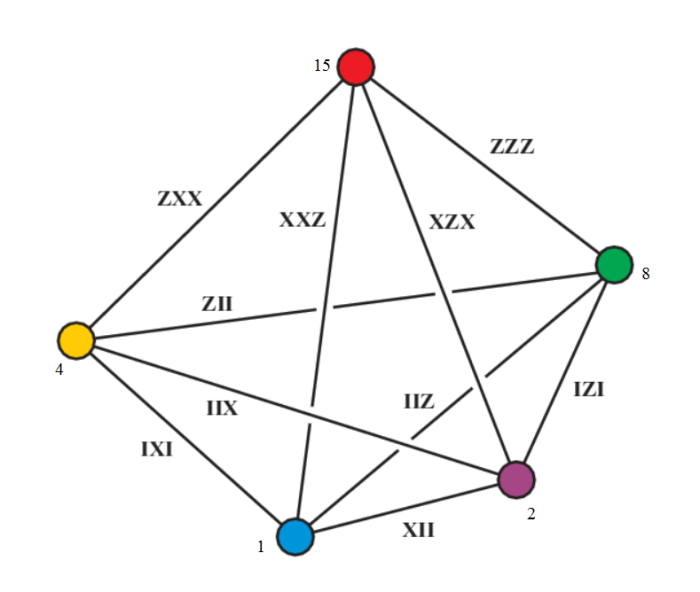
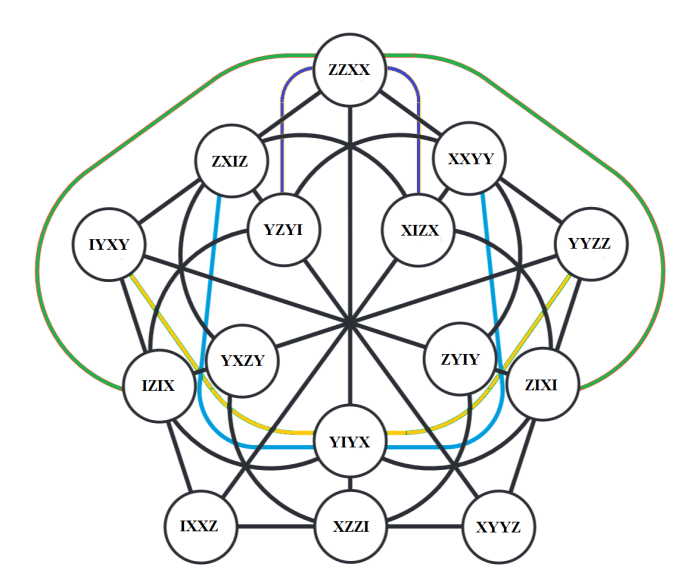
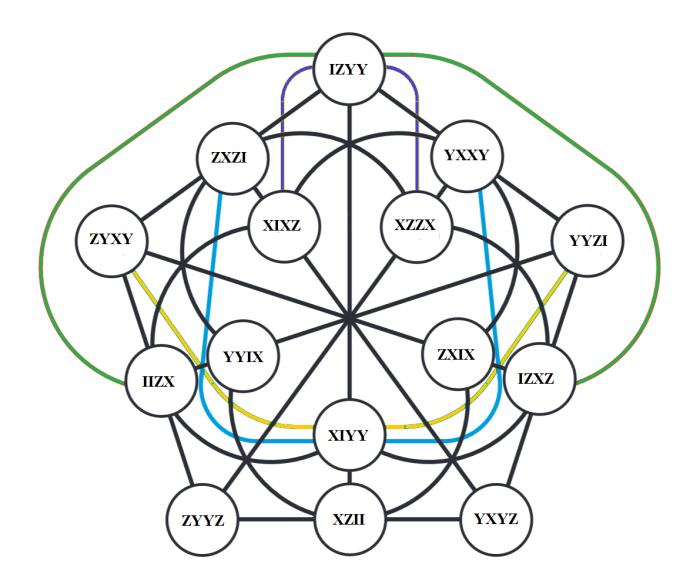
We can organize the 15 fano pencils and 15 fano planes in two doily’s, where a pencil and a plane share the same vertex if they share the same triple of the core of the MVL (figure 11).

FIGURE 13

 

FIGURE 14

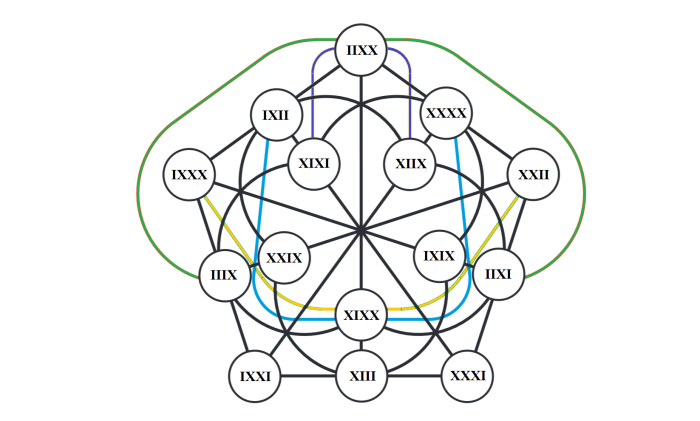
Or, use these two doily’s and the dictionary table in E8 section 15 .

FIGURE 15      15 fano pencils

 

FIGURE 16     15 fano planes

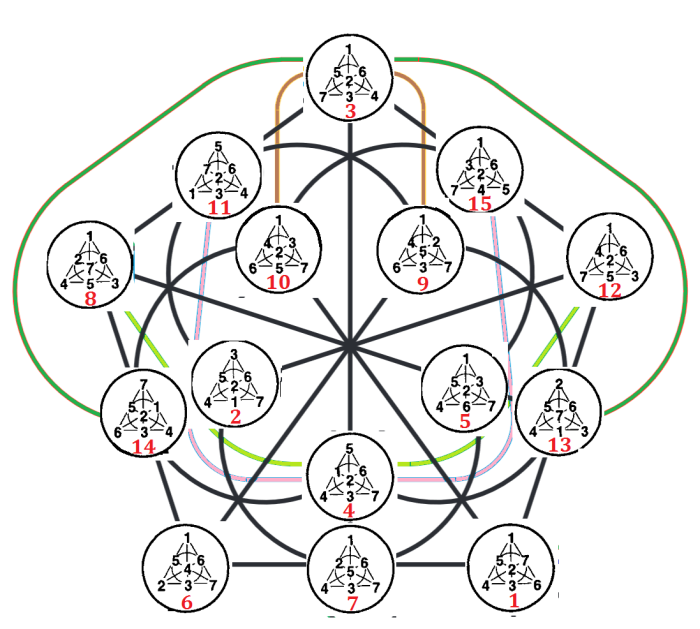
The three lines incident with 7 in each fano are from GQ 78 (the core doily in the MVL). The remaining four lines in each fano become, when translated to 3-qubit operators, the lines of the Mermin pentagrams. The conjugate pentagrams have their lines in the same 5 vertices of the two doily’s. To complete a pentagram, look for the 6 ovoids in the two doily’s and you find the double six of pentagrams with the combination of the 5 corresponding vertices.

GQ 78 admits another doily:

 

FIGURE 17

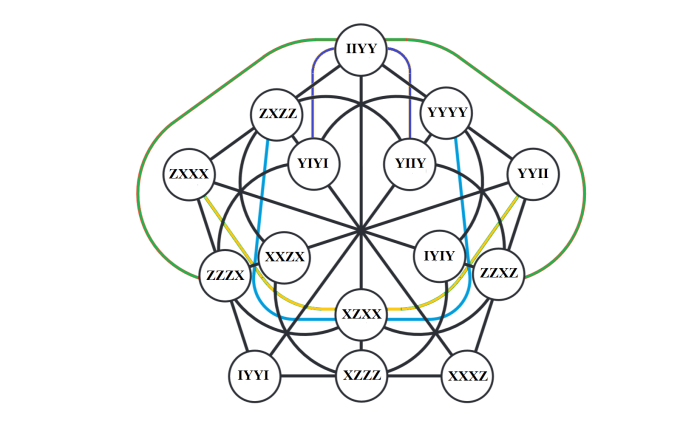
A vertex of the doily represents a fano plane that contains three lines of a flat pencil.

As example, take plane XXZX that shares three lines XIX, XZI and IZX of a flat pencil with plane XXIX and pencil IIZI. In figure 18 you see the dual with XIX, XZI and IZX as points:

 

FIGURE 18

 

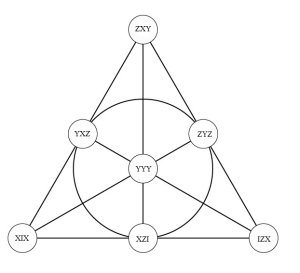
The other four points are in C_YYY . The other 14 all contain YYY and the 3 other triples are easily found from the line of the core by multiplication with YYY.

XXXZ  ↔  XIZ IXZ XXI    YYY    ZYX YZX ZZY

XXZX  ↔  XIX XZI IZX    YYY    ZYZ ZXY YXZ

IIYY    ↔  IXX IYY  IZZ    YYY    YZZ  YII  YXX

XZXX  ↔ ZIX IXX  ZXI    YYY    XYZ YZZ XZY

IYIY    ↔ YIY  XIX  ZIZ    YYY    IYI  ZYZ  XYX

IYYI    ↔ YYI  ZZI  XXI    YYY    IIY  XXY  ZZY

XZZZ  ↔ IXX  XIX  XXI   YYY     YZZ ZYZ ZZY

ZXXX  ↔ IZZ   ZIZ  ZZI   YYY     YXX XYX XXY

YIIY    ↔ XZI  ZXI  YYI    YYY    ZXY XZY  IIY

YIYI    ↔ XIZ  ZIX  YIY    YYY    ZYX XYZ  IYI

ZXZZ  ↔ XIZ  IZZ  XZI    YYY    ZYX YXX ZXY

YYII    ↔ IZX  IXZ  IYY    YYY    YXZ YZX  YII

ZZXZ  ↔ ZIZ  IXZ  ZXI     YYY   XYX YZX XZY

ZZZX  ↔ ZIX  IZX  ZZI     YYY    XYZ YXZ XXY

YYYY   ↔YIY  IYY  YYI     YYY   IYI   YII   IIY

The 15 new triples are antisymmetric and commuting with YYY. They appear in the Witting polytope as a pair of the complex polytope 3{4}2 (red and orange) and a pair of the complex polytope 2{4}3 (green):

 

FIGURE 19

 

The split in these dual polytopes is reflected in the correspondence with a grid and its dual. A grid or GQ(2,1) has 9 points, incident with 2 lines, and 6 lines, incident with 3 points. A 3{4}2 has 9 vertices, incident with 2 triangular edges, and 6 triangular edges, incident with three vertices. But the triples of the edges are not commuting. We must choose the three diagonals and three triangular diameters for the six lines:

YII IYI IIY

YXX XYX XXY

YZZ ZYZ ZZY

YII YXX YZZ

IYI XYX ZYZ

IIY XXY ZZY

And dually… There is more to say about the Mermin squares in a separate page, but now return to the Mermin pentagrams.

The next picture shows a complex polytope 3{3}3 that we recognize as a line of a Mermin pentagram YYY    IIY  XXY  ZZY.

FIGURE 20

 

It is the complement of the line YYI  ZZI  XXI in the plane IYYI. This plane shows as:

 

FIGURE 21

 

Then the plane IIYY with Mermin pentagram line YYY YII YXX YZZ  as a 3{3}3:

 

FIGURE 22

 

And a third one, plane IYIY:

 

FIGURE 23

 

Plane YYYY is special with 6 points on a line:

 

FIGURE 24

 

 

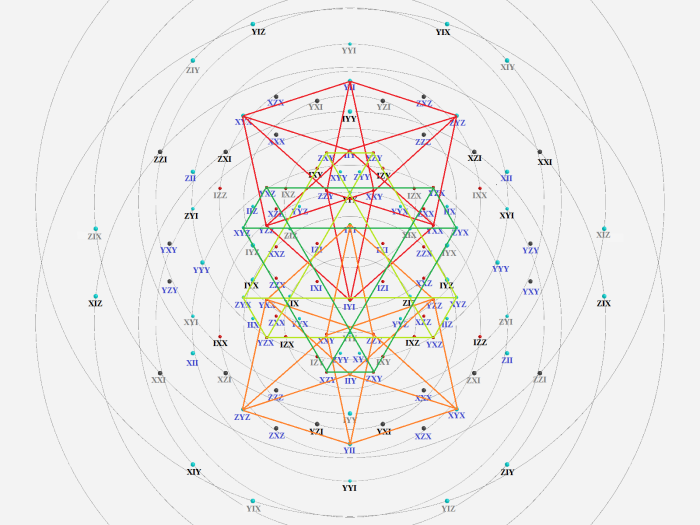
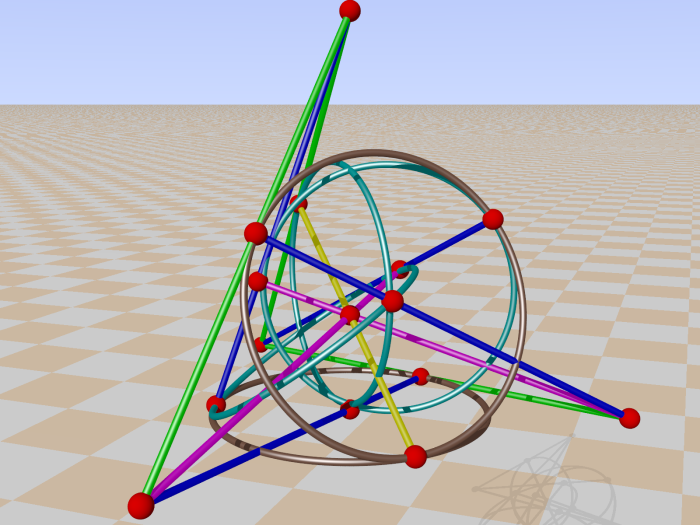
In the Witting polytope the lines of the conjugate Mermin pentagrams give the following pictures:

FIGURE 25     {3} and [3] and DF

We see two complex polytopes 3{3}3 and a hexagram for the common flat pencil. Likewise in the next two pictures:

FIGURE 26     {5} and [5] and BF

FIGURE 27    {6} and [6] and  BD

Mutually commuting qubit operators are related to mutually orthogonal vectors in the Witting polytope.

The other lines of the Mermin pentagrams are not related to complex polytopes, but yet to orthogonal vectors.

FIGURE 28    {12} and [12] and  BE

 

FIGURE 29    {15} and [15]  and   AC

FIGURE 30   {8} and [7]  and   CE

Reflection in the vertical symmetry axis in figure 20 results in {7} and [8] and AE.

 

FIGURE 31   {13}  and [2]   and   AD

FIGURE 32   {2}  and  [13]   and   CD

The yellow hexagrams are part of the core of the MVL. In 3{3}3{3}3 they are 15 tritangents of the 45. They correspond to 15 of the 45 lines of generalized quadrangle GQ(2,4).  The other 30 lines make use of the Schläfli double six.

We can put the conjugate ones in determinant form as

ZZX XYY IXI

XII ZXZ YYX      XXX

YXY IIX XZZ

 

XXZ ZYY IZI

ZII XZX YYZ      ZZZ

YZY IIZ ZXX

 

To see how many pentagrams there are we can make the doily belonging to GQ 68 :

FIGURE 33

 

FIGURE 34

You can read the doily as follows: the set of 15 pencils are IIIZ, IIZI, IIZZ, …. , ZZZI, ZZZZ and the set of 15 planes IIIX, IIXI, …, XXXX . Using the trace map X→1, Z→1, Y→0, I→0, we get two sets of the numbers 1 to 15 in binary notation.

Take for example ZIXI and read this as flat pencil ZIII in plane IIXI, or flat pencil 8  (1000) in plane 2 (0010). . In the picture above the doily you can check that this is the case for two green lines and a blue line ( plane 2 is in point reperesentation 1-4-5-8-9-12-13). Second example: YYZZ is flat pencil 15 (ZZZZ) in plane 12 (XXII). Then ZZZZ•XXII = YYZZ. The two magenta lines and the yellow line in plane 12 (1-2-3-12-13-14-15).

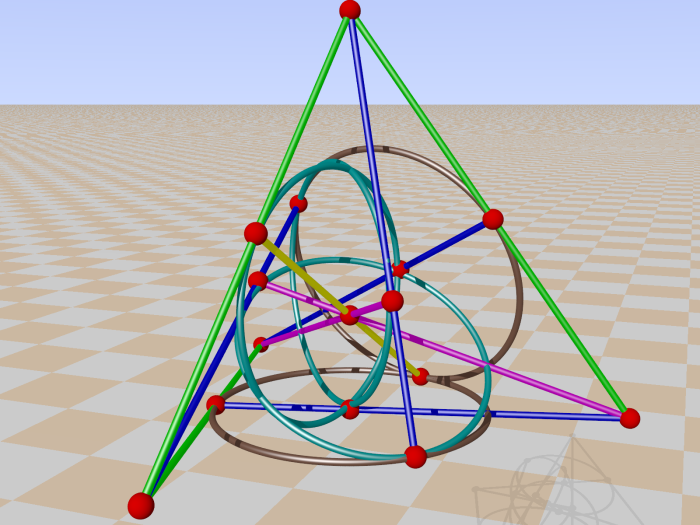
Another GQ is GQ 18 :

FIGURE 35

 

FIGURE 36

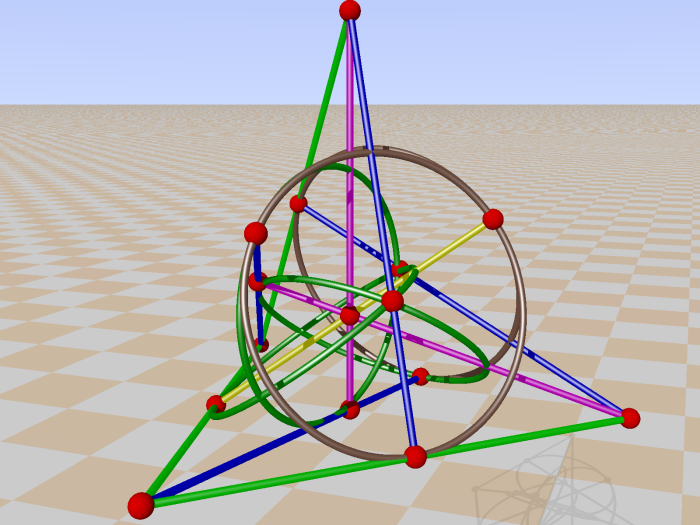
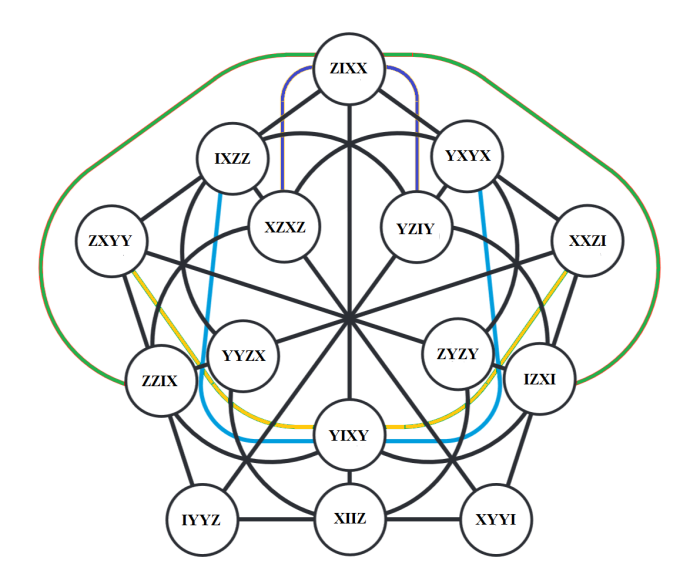
And GQ 28 :

FIGURE 37

 

FIGURE 38

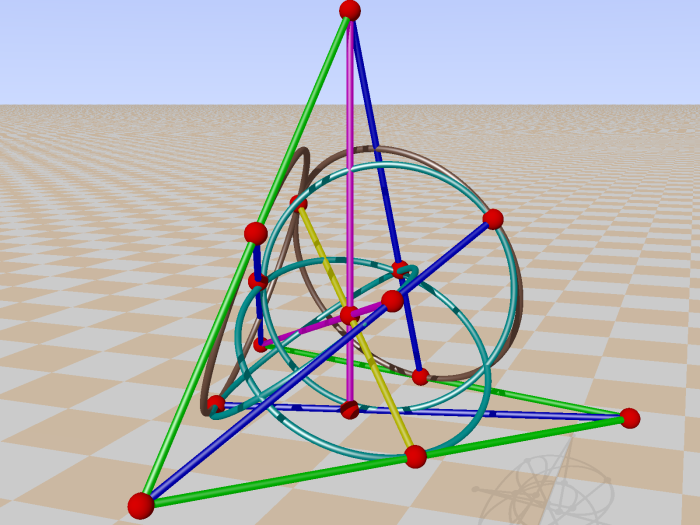
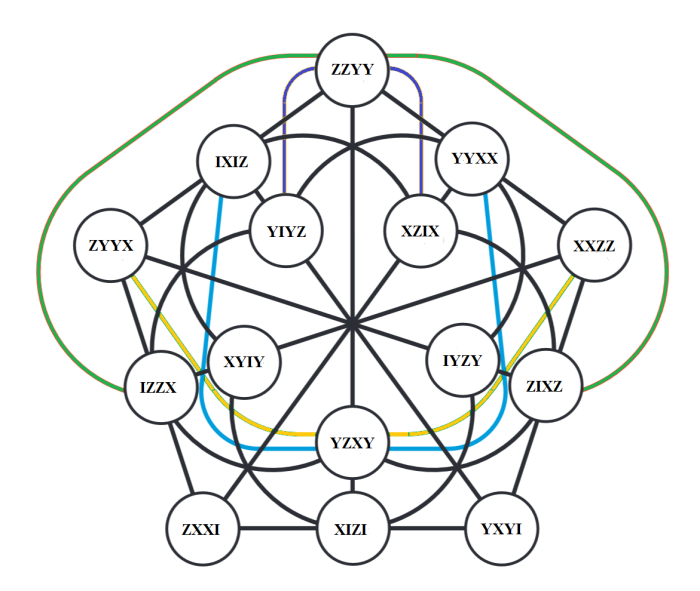
And GQ 38 :

FIGURE 39

 

FIGURE 40

 

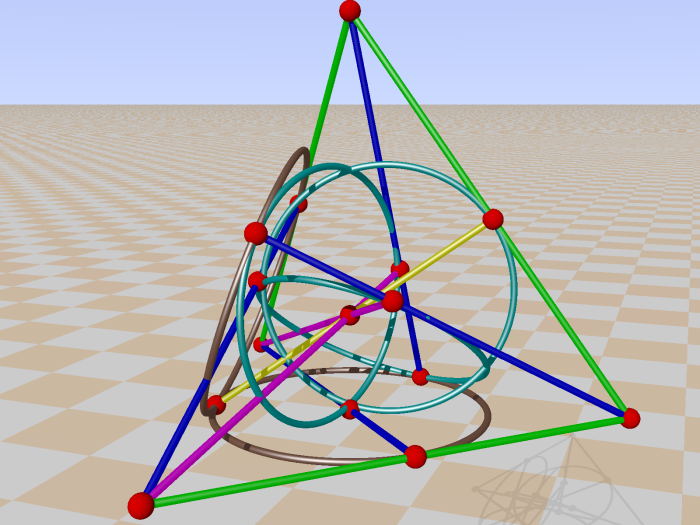
And GQ 48 :

FIGURE 41

 

FIGURE 42

 

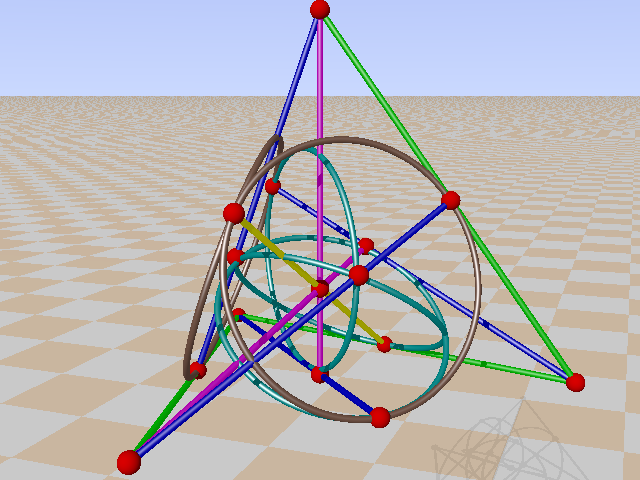
And GQ 58 :

 

FIGURE 43

 

FIGURE 44

The nine PG(3,2)’s from FIGURE 13, 14, 17, 34, 36, 38, 40, 42 and 44 contain the 9×15 = 135 four qubit operators exactly once.

Seven PG(3,2)’s can be summarized in one :

FIGURE 45

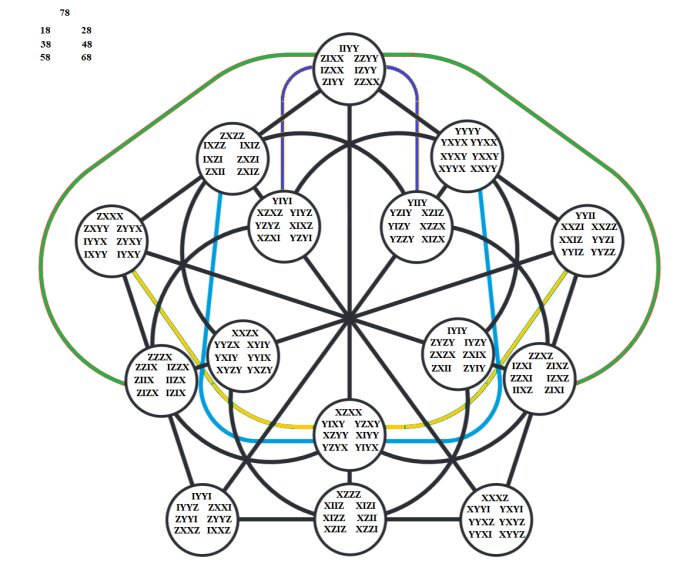
Compare this with FIGURE 16 where in the 15 Fano planes the 15×7 = 105 flat pencils can be recognized in the same vertex. Operators belonging to the same PG(3,2) can be found in the same relative postion within a vertex, indicated in FIGURE 45 in the left upper corner. The 15 planes and 15 full pencils are easily deduced from 78 . For instance IIYY hints to IIXX and IIZZ, and ZXZZ hints to ZIZZ and IXII. In this way FIGURE 45 summarizes the 135 symmetric operators.

 

 

Generalized quadrangle in elliptic space

The points, lines and planes of a projective space can be transformed in points, lines and planes of a elliptic space by choosing a metric. A possible model for such a space is the 3-sphere  where pairs of antipodal points are the elliptic points, lines are great circles and planes are 2-spheres. The result for the GQ(2,2) is below.

The numbering is equal to the GQ at the page about this GQ (paragraph 4 of PG(3,2) ).

The tetrahedron with green edges [ 1 2 3], [ 2 4 6], [1 4 5], [1 8 9], [4 8 12] and [2 8 10] is perhaps helpful to see this.

The plane [1 2 3 4 5 6 7] is the outer sphere (radius 1) with 3 blue meridians incident with point [7]. Only on this sphere is each point pair visible. The other circles are drawn within the sphere with radius 1, so the points outside this sphere are invisible. The sphere with radius 1 becomes the plane at infinity on returning to the projective geometry. The four perpendiculars from vertices [1], [2], [4] and [8] to the oppsite planes complete the 25 straight lines of PG(3,2).

The affine situation of the GQ-page is recovered by double rotation in the 3-sphere or a projective transformation in the projective space.

The points that are drawn seem to be rather chaotic and random, but they actually are  vertices of a 600-cell whose vertices lie on the 3-sphere. Twelve green vertices of a icosahedron, 20 red vertices of a dodecahedron, 12 black vertices of a icosahedron again and 30 golden vertices of a icosidodecahedron. The 120 vertices of a 600-cell are counted as 2×12 of 2 icosahedra, 2×20 vertices of 2 dodecahedra, another 2×12 vertices of 2 icosahedra and 30 vertices of the icosidodecahedron and the 2 poles of which one is visible in the centre of the 2-sphere.

A double 6 configuration can be added:

The points of intersection are vertices of the 600-cell. These are 6 green ones of the icosahedron, 12 red ones of the dodecahedron, 6 black ones of a icosahedron and 6 (double) ones of the icosidodecahedron.

The 15  and 12 lines together are the 27 lines of a GQ(4,2).

PG(3,2) 2

Through each of the 15 vertices is a pencil of 7 lines. Such a pencil of lines has 3 lines in each of the 7 planes through that vertex. And  each line is common to 3 planes. These 7 lines and 7 planes have the combinatorial features of a Fano plane.

Two examples of a pencil of 7 lines:

 

PG(3,2)

All 15 planes of the space are Fano planes.

There are 4 planes like this:

There are 6 planes like this:

There are  4 planes like this:

There is one plane like this:

PG(3,2) and E8

Projection of 240 vertices of the regular polytope representing the 240 root vectors of E8 in the Coxeter plane

 

Smallest projective space with 15 points and 15 planes and 35 lines